Find more notes here : onlineengineeringnotes.com
Unit 2: Hydrostatic Forces on Submerged Surfaces -
Hydrostatics is the branch of fluid mechanics that deals with the behavior of fluids at rest, primarily focusing on the distribution of pressure within a fluid and the forces exerted by a fluid on surfaces submerged in it. Understanding hydrostatic forces on both plane and curved surfaces is fundamental in civil engineering, especially in the design and analysis of structures that interact with liquids, such as dams, tanks, and ship hulls. Here's an overview of the concepts related to hydrostatic forces on these surfaces:
2.1 Concept of Hydrostatics on Plane and Curved Surfaces
2.1.1 Hydrostatic Pressure:
Hydrostatic pressure is the pressure exerted by a fluid at rest due to the weight of the fluid above it. This pressure increases with the depth below the free surface and is described by Pascal's law. Hydrostatic pressure is crucial for understanding how pressure varies with depth in a fluid.
2.1.2 Hydrostatic Force on a Plane Surface:
When dealing with a plane submerged surface (such as the side of a dam or a wall in a water tank), the hydrostatic force on that surface can be calculated using the following key principles:
- The hydrostatic force on a plane surface is directly proportional to the pressure at its centroid.
- The pressure at a certain depth in a fluid is proportional to the depth and the density of the fluid. Mathematically, P = ρ * g * h, where P is the pressure, ρ is the fluid density, g is the acceleration due to gravity, and h is the depth.
To calculate the hydrostatic force on a plane surface, you typically integrate the pressure distribution over the surface area. The formula for the hydrostatic force on a plane surface is:
F = P * A,
where F is the hydrostatic force, P is the pressure at the centroid of the surface, and A is the area of the surface. The pressure at the centroid depends on the depth of the centroid below the free surface.
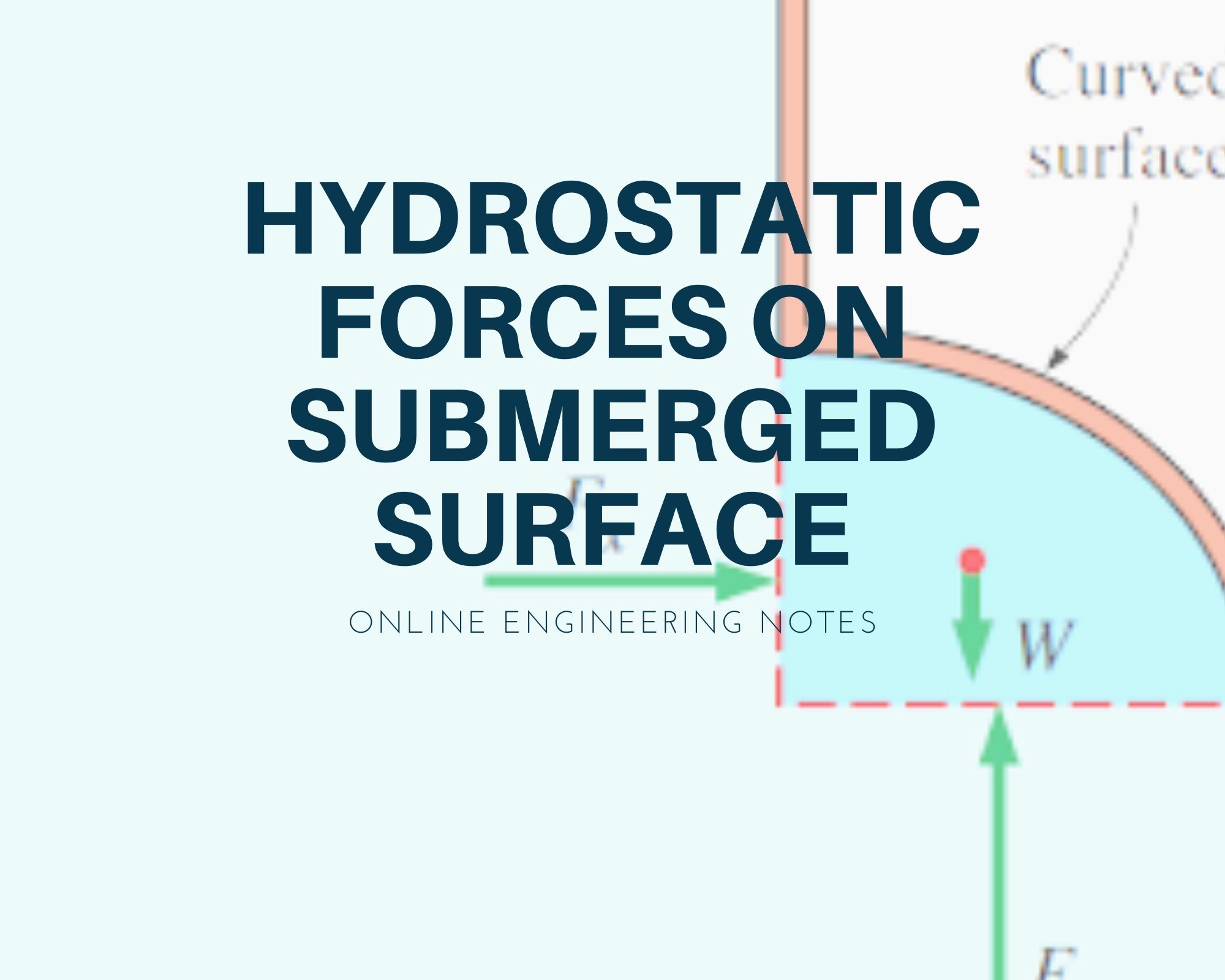
2.1.3 Hydrostatic Force on a Curved Surface:
In the case of a curved or submerged surface with irregular shapes (like a ship's hull or a submerged gate), the calculation of the hydrostatic force becomes more complex. The key principles to consider are:
- Pressure distribution varies across the curved surface, and the pressure at each point is determined by the depth and orientation relative to the free surface.
- The hydrostatic force on a curved surface is calculated by integrating the pressure distribution over the entire surface area.
The pressure at any point on the curved surface is determined using the same principles of hydrostatic pressure (P = ρ * g * h). The total hydrostatic force on the curved surface is the sum of the pressure contributions from all points on the surface.
In practical engineering applications, these calculations can be complex and may require the use of numerical methods or computational tools to obtain accurate results.
Understanding the concepts of hydrostatic forces on plane and curved surfaces is essential for civil engineers when designing and analyzing structures that interact with fluids. It helps ensure the stability and safety of various hydraulic structures and systems, and it is a fundamental aspect of fluid mechanics in civil engineering.
2.2 Total Pressure and Center of Pressure
2.2.1 Total Pressure:
Total pressure, also known as hydrostatic pressure, is the sum of the hydrostatic pressure and the dynamic pressure at a given point in a fluid. It represents the total energy at that point. In the context of hydrostatics, we typically focus on hydrostatic pressure, which is the pressure due to the weight of the fluid above a point in the fluid column.
Total pressure varies with the depth below the free surface of the fluid, and it is critical in determining the forces on submerged surfaces. Total pressure is expressed as:
Total Pressure (P_total) = Hydrostatic Pressure + Dynamic Pressure
P_total = P_hydrostatic + P_dynamic
P_total = ρ * g * h + 0.5 * ρ * V^2
Where:
- P_total is the total pressure.
- P_hydrostatic is the hydrostatic pressure due to fluid weight.
- P_dynamic is the dynamic pressure due to fluid velocity.
- ρ is the fluid density.
- g is the acceleration due to gravity.
- h is the depth below the free surface.
- V is the velocity of the fluid.
2.2.2 Center of Pressure:
The center of pressure (CP) on a submerged surface is the point where the resultant hydrostatic force can be considered to act. It is a critical concept in hydrostatics and is essential for stability analysis and design of submerged structures. The location of the center of pressure depends on the shape and orientation of the surface.
Center of Pressure on a Horizontal Plane Surface: For a horizontal plane surface submerged in a fluid, the center of pressure is located at the geometric center of the surface. This means that the resultant hydrostatic force acts vertically upward through the centroid of the surface.
Center of Pressure on a Vertical Plane Surface: On a vertical plane surface, the center of pressure is at a depth (h_CP) below the free surface. The depth is determined by the shape of the surface and can be calculated using specific formulas. For example, for a vertical rectangular surface, the center of pressure is located at h_CP = (2/3)h from the free surface.
Center of Pressure on an Inclined Plane Surface:The center of pressure on an inclined plane surface depends on the orientation of the surface. For an inclined plane surface, the center of pressure is below the free surface, and its location is determined by the angle of inclination and the shape of the surface. Formulas are available to calculate the position of the center of pressure for different cases.
Center of Pressure on a Curved Surface: Calculating the center of pressure on a curved surface is more complex and typically requires integration of the pressure distribution over the entire surface. The center of pressure for a curved surface depends on the shape of the surface and the depth of immersion.
Understanding the concept of center of pressure is crucial for assessing the stability and equilibrium of submerged structures and for ensuring that forces and moments are properly considered in the design process. It allows engineers to determine the point where the hydrostatic force can be applied to analyze the structural response and stability.
2.3 2.3 Pressure diagram (horizontal, vertical and inclined plane and curve surfaces)
2.4 Computation of pressure forces on gates, dams, head water tank and other hydraulic structures (plane and curve)
Computing pressure forces on gates, dams, headwater tanks, and other hydraulic structures is a crucial aspect of civil engineering and fluid mechanics. These calculations help engineers design and analyze these structures to ensure their stability and performance. Here's an overview of how to compute pressure forces on both plane and curved surfaces in the context of various hydraulic structures:
Computation of Pressure Forces on Plane Surfaces:
1. Dams: Dams are commonly designed as inclined plane surfaces that retain water. The pressure force on the dam can be calculated using the hydrostatic pressure equation and integrating it over the dam's surface. The formula for the hydrostatic force on a dam can be written as:
F = P_avg * A
Where:
- F is the total hydrostatic force.
- P_avg is the average pressure on the dam's surface.
- A is the area of the dam's surface.
To find P_avg, you need to calculate the centroid of the pressure distribution on the dam surface, which varies with depth. Then, integrate the pressure over the surface area.
2. Gates: Pressure forces on gates depend on the shape, orientation, and depth of the gate. The pressure distribution on the gate can be calculated using the hydrostatic pressure equation. You'll need to compute the pressure at various points on the gate and integrate to find the total force.
3. Headwater Tanks:The pressure force on the walls of a headwater tank is determined by the depth of water, the shape of the tank, and the orientation of the surfaces. You can calculate the total force by integrating the pressure distribution over the tank walls.
Computation of Pressure Forces on Curved Surfaces:
1. Dams with Curved Spillways: Some dams have curved spillways. To calculate the pressure force on these curved surfaces, you need to consider the shape of the surface and the pressure distribution. The process involves dividing the curved surface into small elements and finding the pressure on each element. Then, integrate the forces over all the elements.
2. Curved Gates or Submerged Objects: For curved gates or other submerged objects, pressure forces depend on the geometry and orientation of the object. You may need to break the surface into smaller elements and calculate the pressure force on each element. Then, sum up these forces to obtain the total force on the curved surface.
In practice, these calculations can become complex, especially for irregular shapes and curved surfaces. Engineers often use numerical methods and computational fluid dynamics (CFD) simulations to compute pressure forces accurately in real-world hydraulic structures. These methods allow for detailed modeling of the pressure distribution, making it possible to account for variations in depth, shape, and orientation.
Additionally, keep in mind that buoyancy forces may also need to be considered, especially for structures that float or partially submerge. Proper consideration of pressure forces is crucial to ensure the structural integrity, safety, and stability of hydraulic structures like dams, gates, and headwater tanks in various civil engineering applications.